Question: Derive a relation between angular and linear displacements. OR Show that s = rθ.
ANSWER
When a boy goes to school on bicycle, the wheels of bicycle rotate and at the same time covering a linear displacement on the road. So there must be a relationship between angular and linear displacements.

Consider the figure to the right. Let the particle at A starts moving on a circle and reaches point B. Let the length of the arc AB = radius of the circle = r By definition

Take another point C on the arc AB. Let AC = s, and
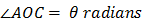
Now we know that the angle subtended at the center of the circle depends on the length of the arc; greater the arc greater the angle and vice versa. Therefore, for two angles, the ratio of their arcs is equal to the ratio of the angles.

Here s is the linear displacement and θ is the angular displacement. So for constant radius, the angular and linear displacements are proportional. This is the required relation.

Pingback:angular-motion-different-parameters-of-angular-motion – msa