Question 5: What is projectile motion? Give examples. Derive mathematical equations for maximum height attained, time of flight and range of projectile.
State in which conditions the range will be maximum if speed of projection is kept constant in a uniform gravitational field?
Answer
Projectile Motion
Projectile motion is the two-dimensional motion under the action of gravity.
Explanation
Consider a ball thrown horizontally from a certain height from the ground. The ball travels forward horizontally as well as falls vertically downward. The ball moves in two directions simultaneously. Such a motion is called projectile motion.
| Can you think of a better example of projectile than a basketball scored from a distance?Well, a basketball scored from some distance, say one-fourth of the ground, is a perfect example of projectile motion. The ball follows a parabolic trajectory well judged by the scorer player. |
Let’s consider an object is thrown upward with some angle θ with the horizontal direction such that 0°<θ<90°.
The following characteristics of the motion can be noted.
- The horizontal component of the velocity of the projectile remains constant throughout the flight.
- The vertical component of the velocity Vy decreases with a uniform rate under the action of gravity.
- The vertical component of the velocity becomes zero at the highest point.
Now to study the motion of the projectile, we resolve its acceleration along horizontal and vertical directions which are independent of each other.
Horizontal component of the acceleration ax = 0
Vertical component of the acceleration = ay = -g
(g is directed downward)
After any interval of time t, the final velocity along horizontal direction
![]()
(vi being the initial velocity of the projectile)
Along vertical direction, the component of velocity,
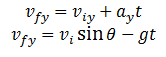
Magnitude of velocity:
Hence the magnitude of velocity after time t is,
![]()
Direction of velocity
Its direction can be calculated by finding the tangent of the angle θ.
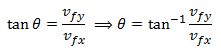
Maximum Height
In order to find the maximum height of the projectile, H, we use third equation of motion.
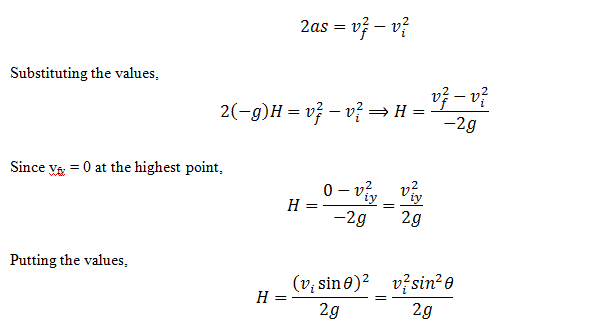
This equation gives the maximum height of projectile.
Time of flight
Maximum time of flight from the point of projection to the point of impact (at the same height) can be calculated by using 2nd equation of motion.
![]()
As flight is a vertical phenomena, therefore, at the strike point when the body reaches the same height, s = 0. If time of flight is
t = T
vi = viy = visinθ
a = -g
Put these values in (X)
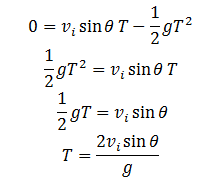
If we are interested in the time T’ when the projectile reaches the maximum height H, then it is equal to half of the total time of flight.

Range of Projectile
Range is the maximum distance the projectile covers in the horizontal direction.
In order to determine the range R of the projectile, we use the equation
s = t —— (Y)
Where s = R and is the average velocity.
Now
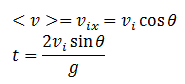
By substituting theses value in (Y)

Since 2sinθcosθ = sin2θ, therefore,
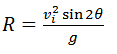

Pingback:index-long-questions-ch3 – msa